Persistent identifier with citation: Harris Cline, Diane. “The Social Network of Socrates.” CHS Research Bulletin 7 (2019). http://nrs.harvard.edu/urn-3:hlnc.essay:ClineD.The_Social_Network_of_Socrates.2019
To enlarge a figure, click directly on the image.
The spreadsheet referred to below is available to download.[1]
In recent years Social Network Analysis has become a tool for analyzing a remarkably wide range of social networks, not just inside Social Media (Facebook, Twitter) but in every imaginable setting.[2] There are several reasons for ancient historians to study social networks. We are interested in the generative patterns that begin a network from the first pair of relations, we are interested in better understanding why some ideas are diffused through networks and others don’t catch on. We are interested in the structure of the network and its sub-communities, and for history, we want to better understand the social relations of the time period of our specialty. At a macro level, we are curious about how new ideas and changes percolate through and ultimately transform a society enough so that we give an era or period of time a new name.
How to build a social network for historic biography
One thing Social Network Analysis does very well is to identify clusters inside a larger group. I use Social Network Analysis to understand the various communities in mid-fifth century Athens and see who Socrates knew and find those to whom he had the closest links. Suspend for the moment the issues of whether Plato’s dialogues are fictional or factual, and whether all of these people actually existed. This may be a study of the social network of Plato’s imaginary construct of Socrates rather than the actual mid-fifth century Socrates. This is an experiment to see if the world painted by Plato holds together. The social world of Socrates as reported by Plato does have cohesion.
Social Network Analysis maps and measures the fabric of society, revealing the subsets within the whole and allows us to identify who held the most prestige, or who served as hubs, brokers, bridges, or connectors between the clusters inside the network. The first step in the method is to assemble the sources from which to build the data base. For Socrates’s social network, I decided to use all of Plato’s dialogues and letters. To begin, I looked for mentions of individuals who had social relations with Socrates, and with each other.
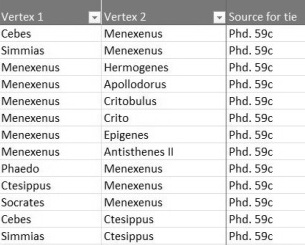
In a spreadsheet, I recorded these relationships, noting who the pairs were, the citation, what city or village they were from, and what their role was in Socrates’s life at the time of the dialogue – if they were acquaintances, students, or fellow philosophers, or parents of students, and so on. The minimum one needs to record is simply the two names in the relationship, but the documentation is important for justifying and re-investigating those relationships later. I collected this information by going page by page through the dialogues of Plato, collecting pairs of names of people tied to each other socially, and recording them in a spreadsheet with two columns. I then looked up each person in Debra Nails, The People of Plato [3] to be sure I had disambiguated those who shared the same name. The spreadsheet with these pairs of names who have some type of relationship is called an “edge list” in Social Network Analysis, and is the backbone of the analysis (fig. 1).[4]
To demonstrate the method for collecting the pairs of names, let’s walk through one short text, Xenophon’s Symposium.
Late one afternoon, some 2450 years ago (421/420 BC), Socrates was walking down a street in Athens with some friends, including students and their family members. Approaching them was another cluster of people Socrates knew, including Callias, one of the richest men in Athens, who had invited a philosopher from other city to come give some lectures and meet his sons and their fellow students. When they get close, Callias stops and invites Socrates to join him for dinner and a symposium. Reluctantly at first, Socrates and his companions finally join Callias’s group and they all head off together The result? Xenophon’s Symposium records it. As we look at the first few paragraphs, I would ask you to imagine in your mind the edge list you would have to make to record these relationships.
The occasion was a horse-race at the great Panathenaic festival. Callias, the son of Hipponicus, being a friend and lover of the boy Autolycus, had brought the lad, himself the winner of the pankration, to see the spectacle. As soon as the horse race was over, Callias proceeded to escort Autolycus and his father, Lycon, to his house in the Piraeus, being attended also by Niceratus. But catching sight of Socrates along with certain others (Critobulus, Hermogenes, Antisthenes, and Charmides), he bade an attendant conduct the party with Autolycus, whilst he himself approached the group, exclaiming: “A happy chance brings me across your path, just when I am about to entertain Autolycus and his father at a feast. The splendour of the entertainment shall be much enhanced, I need not tell you, if my hall should happily be graced by worthies like yourselves, who have attained to purity of soul, rather than by generals and cavalry commanders and a crowd of place-hunters.” Whereat Socrates: “When will you have done with your gibes, Callias? Why, because you have yourself spent sums of money on Protagoras, and Gorgias, and Prodicus, and a host of others, to learn wisdom, must you pour contempt on us poor fellows, who are but self-taught thinkers in philosophy compared with you?” “Hitherto, no doubt” (retorted Callias), “although I had plenty of wise things to say, I have kept my wisdom to myself; but if only you will honour me with your company to-day, I promise to present myself in quite another light; you will see I am a person of no mean consideration after all.” Socrates and the others, while thanking Callias politely for the invitation, were not disposed at first to join the dinner party; but the annoyance of the other so to be put off was so obvious that in the end the party were persuaded to accompany their host. After an interval devoted to gymnastic exercise (and subsequent anointing of the limbs) by some, whilst others of them took a bath, the guests were severally presented to the master of the house. Xenophon, Symposium 1.1-8 [5]
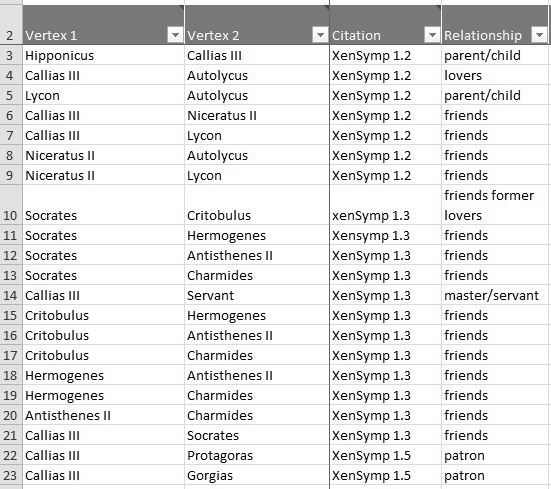
From these passages we can develop a good start on the edge list. Figure 2 shows the beginning of the edge list for just the first three paragraphs of what we just read.
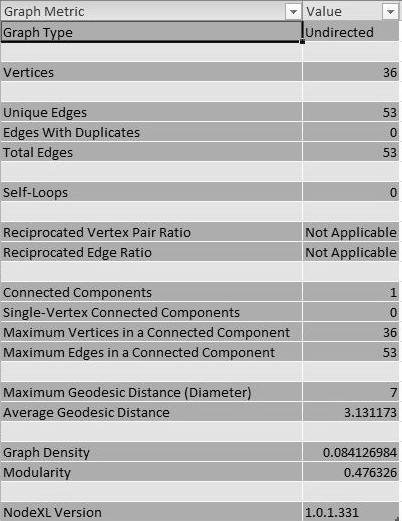
Once the edge list is complete, we cut and paste the pairs of names (two columns) into the SNA program. In this case, using NodeXL, we can see the overall metrics for the network (Figure 3). For all of Xenophon’s Symposium, we have 36 actors, 53 edges, in one connected component. The latter means everyone is connected in some way within the network, with no dyads or triads off on their own. The network metrics also tell us that the network has a maximum geodesic distance of 7, and an average diameter of 3.13. This means that the most peripheral nodes are a distance of seven hops from each other, but on average it takes only about three hops (3.13) for people to find each other or for information to diffuse through the network.
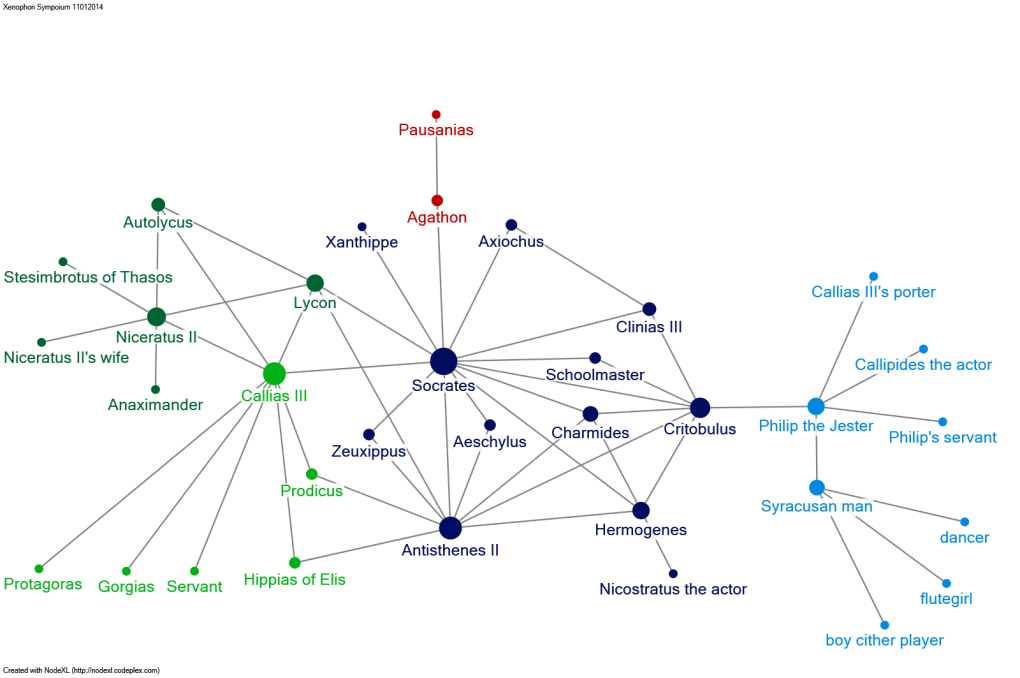
This excerpt from Xenophon’s Symposium illustrates several features of social network analysis. The two groups that meet on the street are clusters, and when they merge at Callias’s house, they merge into one connected component. In Figure 4 above, we have the composite network for all of Xenophon’s Symposium. The size of the nodes are proportional to the number of ties (or “degree centrality measure”) of each actor. The colors signify which cluster individual actors belong to. Starting from the center of the chart, we have the large dark blue cluster around Socrates, bounded by Antisthenes on the bottom, Zeuxippus to the left, and Critobulus on the right. The second cluster in green is focused on Callias, located directly to the left of Socrates, and whose cluster includes the five nodes below him. A third cluster has a focal point on Niceratus I, one of the guests, situated to the upper left of Callias in dark green. Niceratus I is the center of a six-point star, two of which are Callias and Lycon. Both of these have direct ties to Socrates, but Niceratus does not, thus he is two degrees of separation away from him, dependent on a path that goes through either Lycon or Callias. The evening’s entertainers form their own cluster on the far right of the sociogram in light blue, with Critobulus and Philip the Jester acting as the bridges. That cluster has eight members, but the Syracusan man and Philip the Jester play the most important roles in the network, connecting three actors each.
Now is the time to discuss boundaries for our networks and how to discern whether a person belongs in the data set or not. All ties are not equal. Some only met at the party, others are family members, yet others are students of Socrates. Because of the variety of ties, we would call this a multiplex network. While Socrates and Callias and their friends meet the performers, they probably do not maintain an enduring relationship with them. Hard decisions have to be made. This is an ego-network for the purpose of discovering the social network of Socrates. Are the relationships with the evening’s entertainers (hired by Callias and present in his house) really part of Socrates’s social network? Are they meaningful relationships or are they merely fleeting interactions? Such judgment calls must be based on the purpose of the study: If the purpose of the Social Network Analysis is to map everyone who appears in Xenophon’s Symposium we ought to include them, but if we are using this text as one of many sources for the purpose of looking for those who were closest to Socrates or who are at least a part of his personal ego-network, we might choose to exclude them.
This is the method for building the edge list.[6]
Results
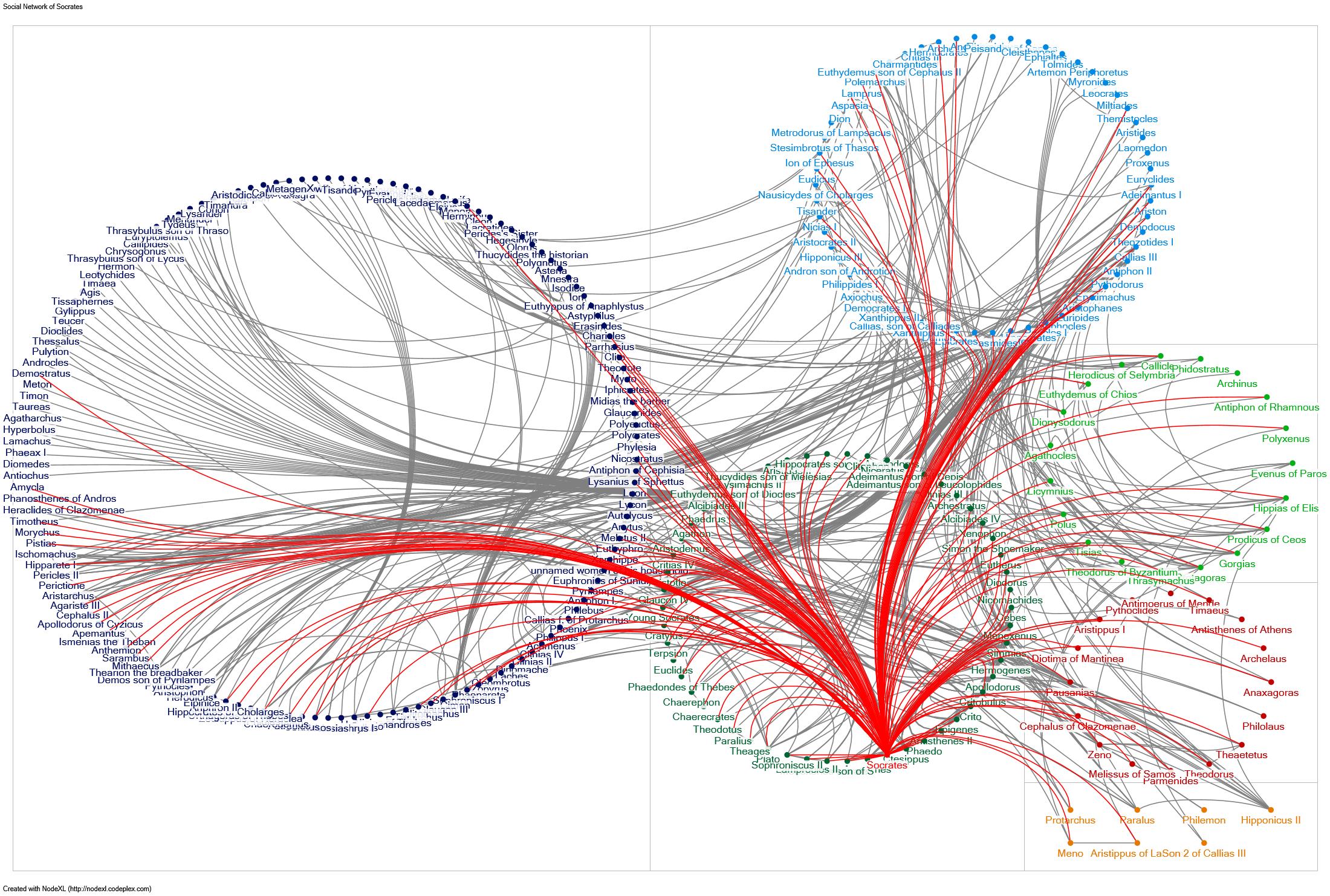
For the whole network that I built using all of Plato, we get an edge list that has 481 rows, or relationships, among 186 people. Socrates is at the core, and where we see red lines we have a one-hop or first degree relationship between him and his acquaintance. Below we have some of the most well-known people and their ties highlighted in the .gif. Notice the clustering as we go around the network. Philosophers, sophists, and people who appear in the dialogues as interlocutors tend to be on the left side and top. The Socratics are situated more on the right and below Socrates.
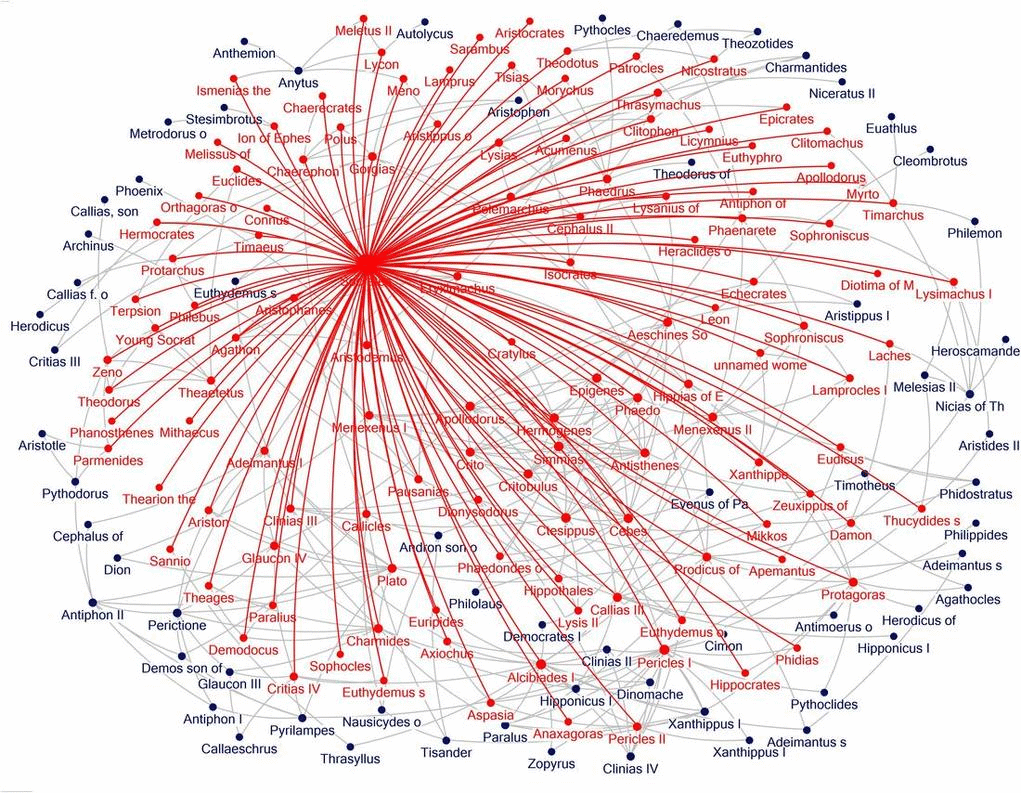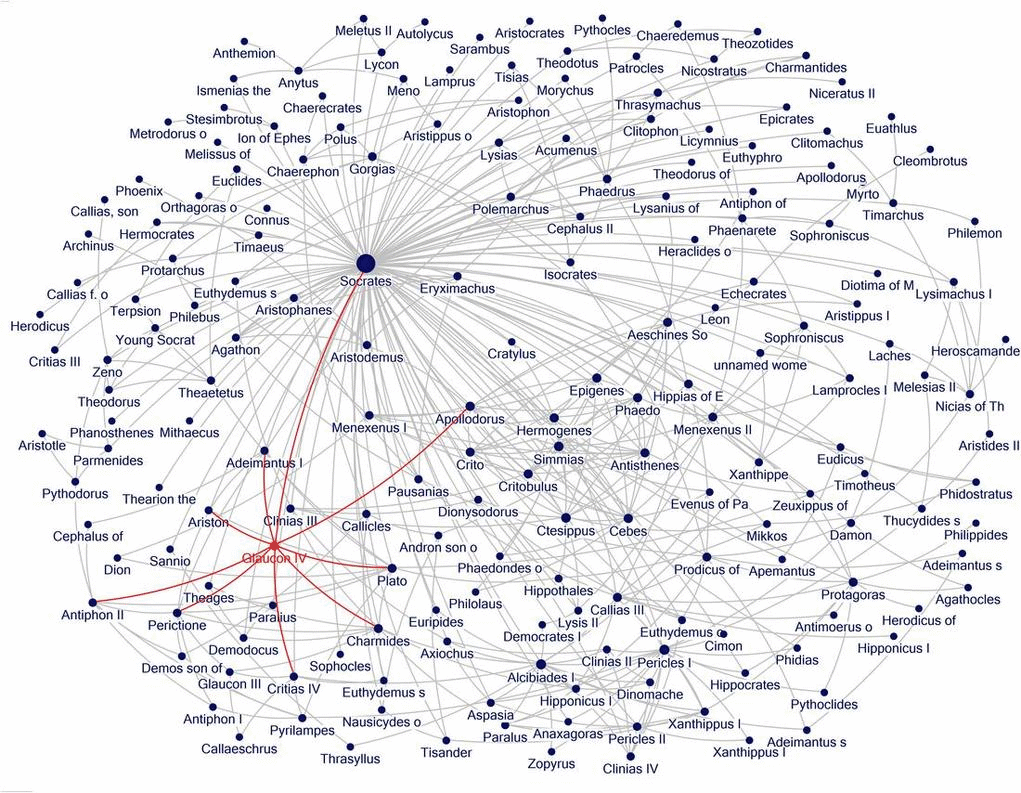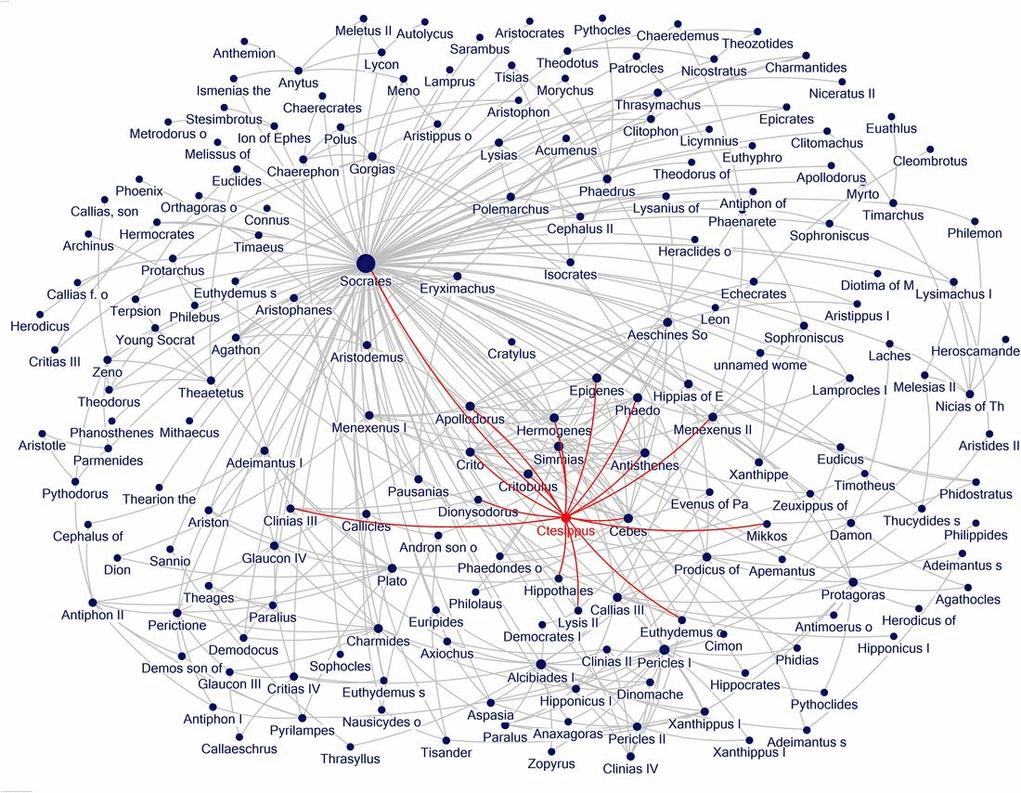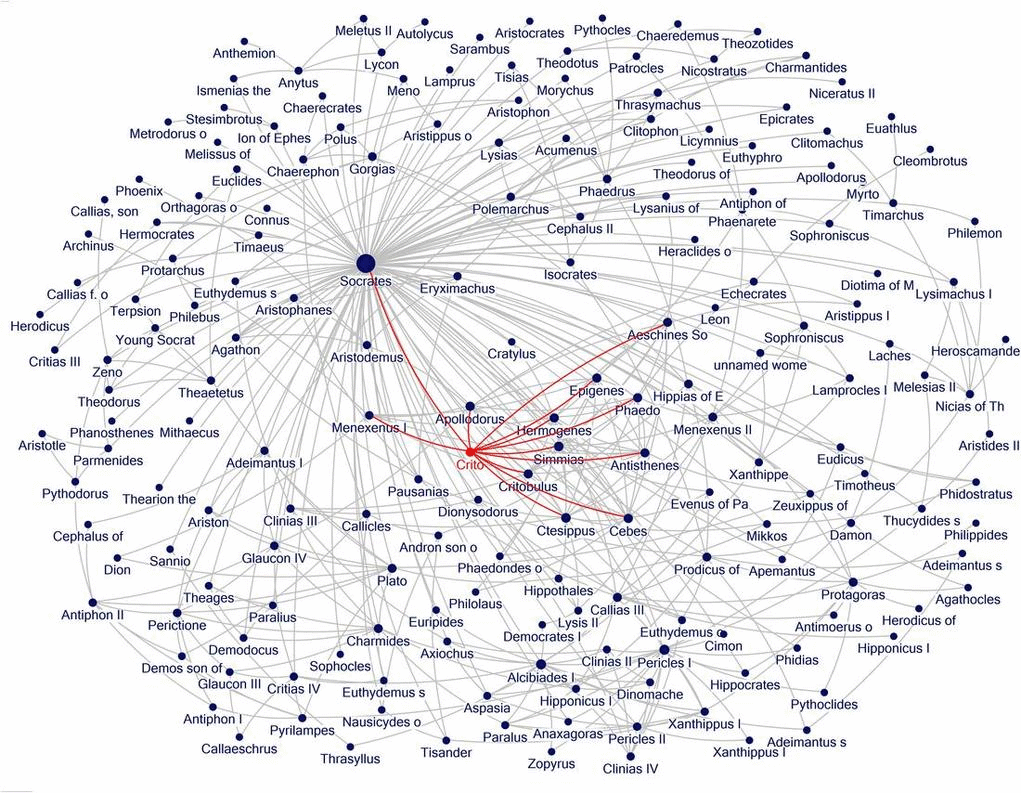
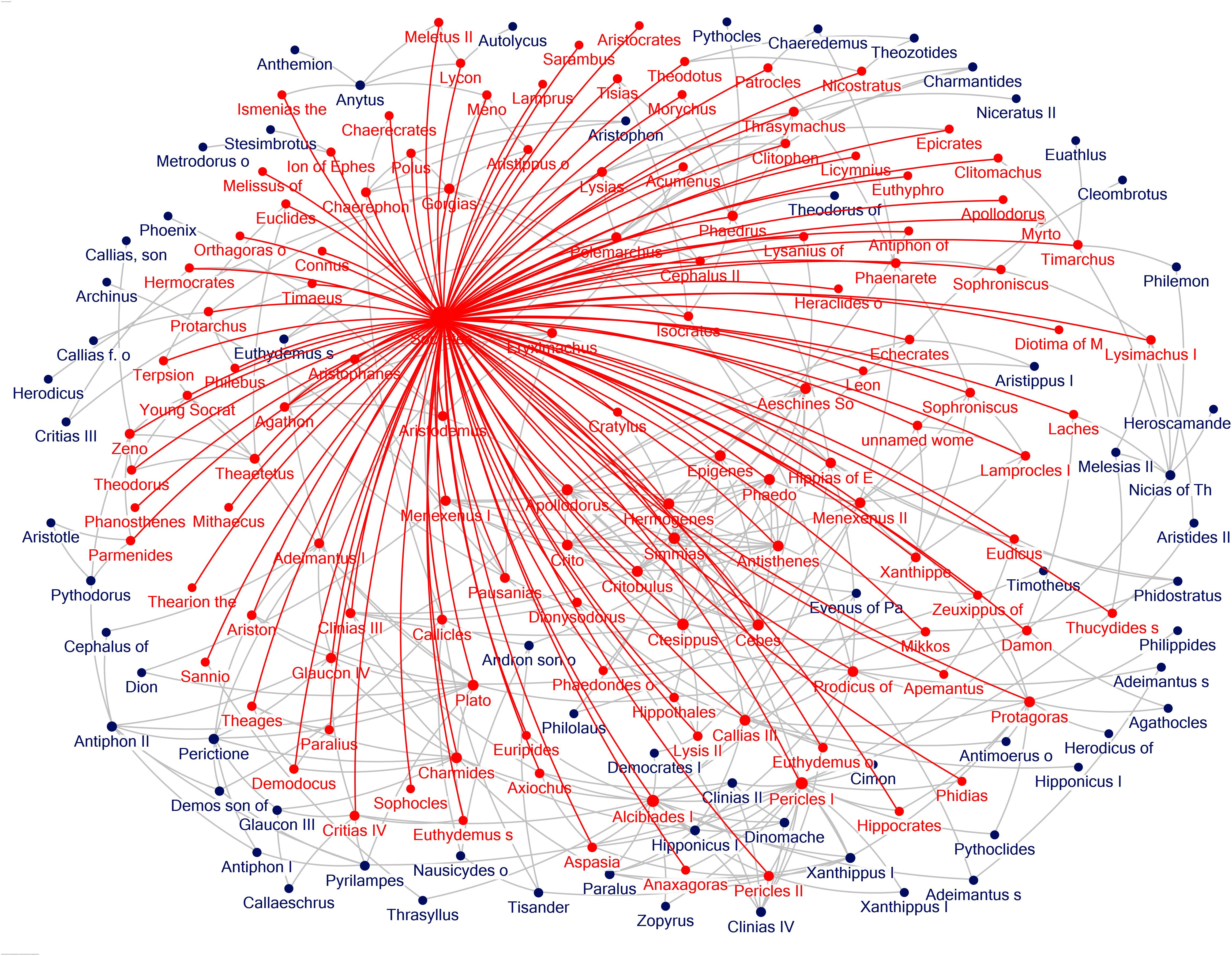
Here in Figures 5 and 6 we are looking at the network using a layout algorithm that lets us explore the core and periphery of the network. The edges for Socrates’s first degree relations are in red (fig. 6), and he has 120 direct relationships. There are a few nodes that form a third degree out from Socrates, visible by looking at a first degree node in red, then tracing the ties out to a blue node that must be a second degree at least. One example is Heroscamander on the far left side, connected to Socrates through Laches and Nicias of Thebes.
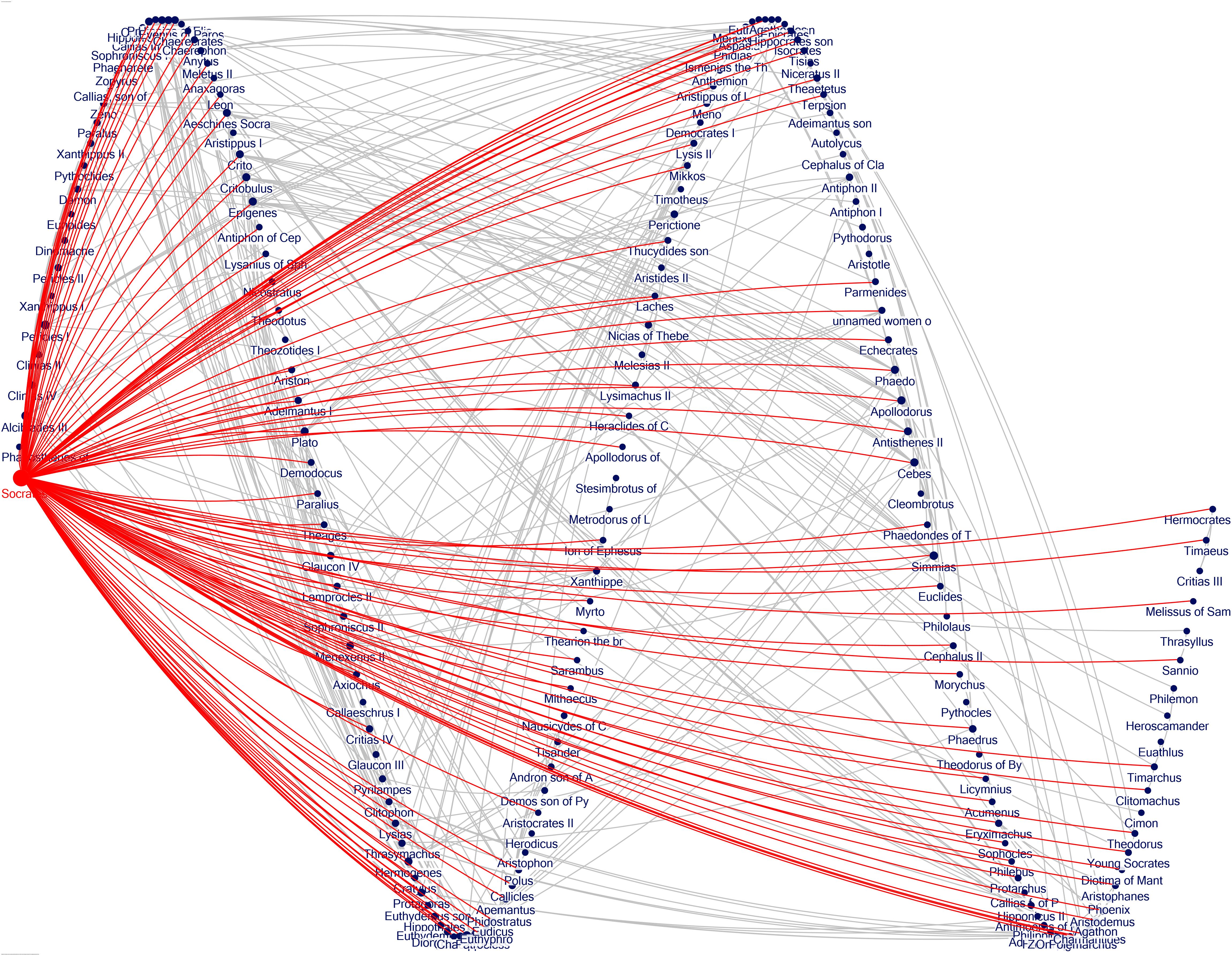
In Figure 7, we have a view of the network using a sine-wave layout, again with Socrates lit up in red. This lets us get a feel for the density and cohesion of ties. Looking for the nodes which do not have red edges, we discover the second degree people, who appear in the dialogues as a friend of a friend from Socrates’s perspective.
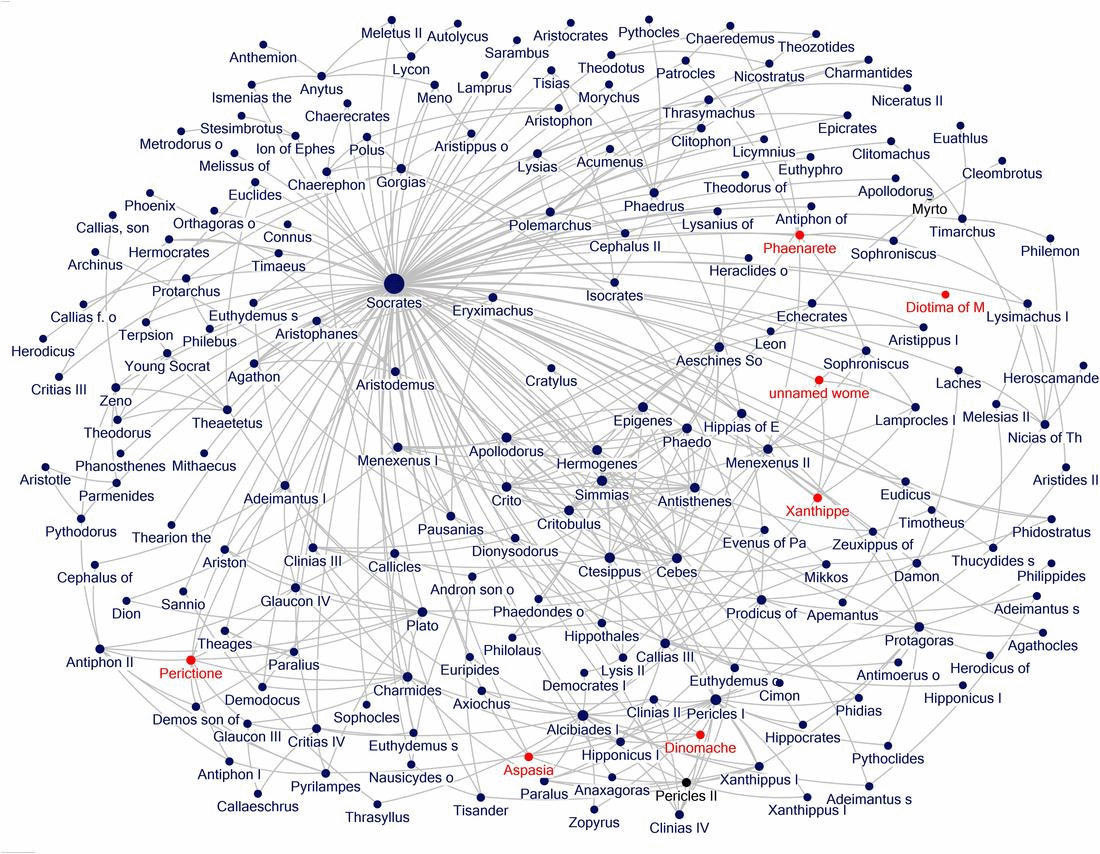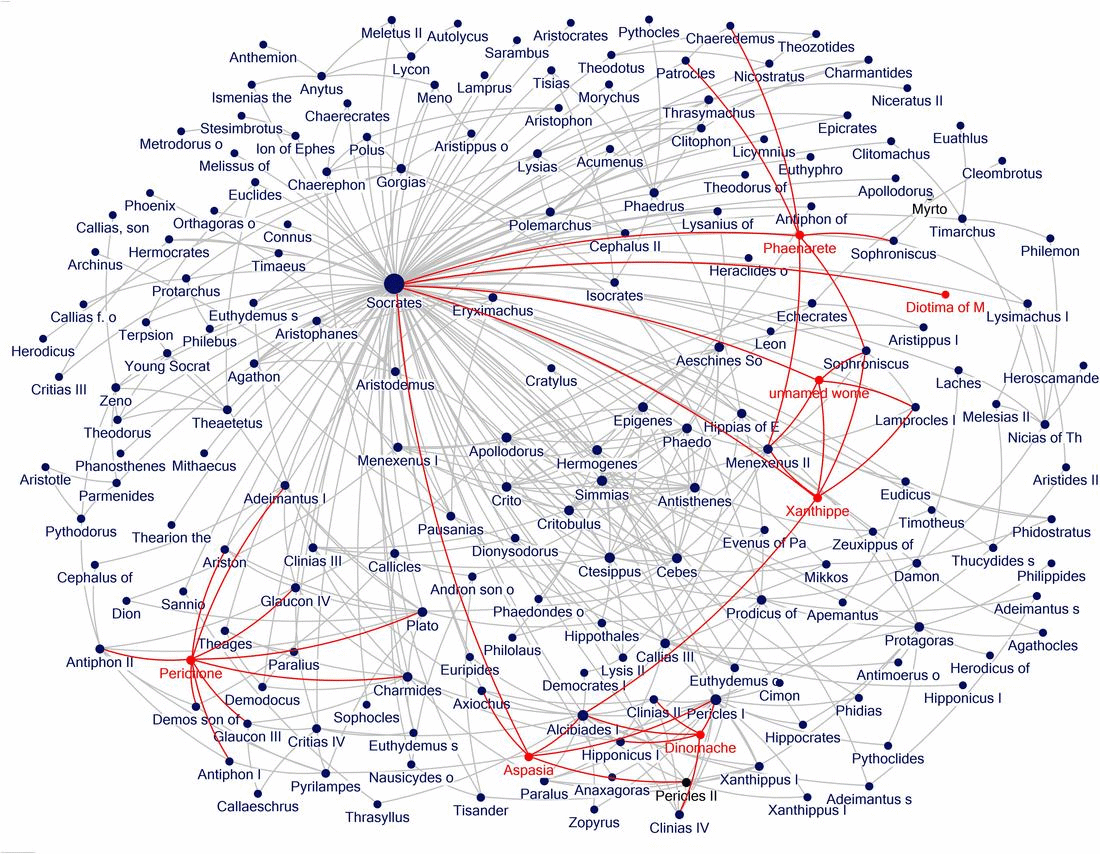
Women are not common in Socrates’s network, in fact there are only seven (although one of those is “unnamed women in Socrates’s household”). We can demonstrate this through using colors. In Figure 7, blue is used for men and red for the women, so that we can quickly identify their positions in the network. When as a group they are lit up, we see them stretched across the network, connected to each other (with the exception of Perictione). Here might be a good place to mention that I included Diotima, and probably others who may not be real people, but she appears isolated and her presence is not skewing our results in any significant way.
Metrics
In addition to the visualizations, in NodeXL and in all social network analysis programs, we get some measurements for the network as a whole, and each individual inside it. We learn, for example, that the average geodesic distance of the network is six, which means that at the maximum it takes six hops from the origin to another node at the periphery. Another way to put it is that at most there is six degrees of separation. The average is under three hops, though, calculated at 2.6. This is a very close group, also called “a Small World.”
We are able to look at the scores for every individual in the network. The program calculates each node’s position and relative structural usefulness in terms of their Degree, Betweenness centrality, and Eigenvector centrality in addition to other measures. Now we will go through each of these to see what they mean.

NodeXL presents two panes simultaneously, the data and the visualization. Figure 9 has each person in a row with their scores. The first darker shaded column in the left window gives a person’s Degree score (number of neighbors), and if we look at the row with the statistics just for Socrates (row 3), it tells us how many ties Socrates has, 121. Remember that in the whole network we have 481 edges, so his acquaintances also know each other. The next column shows us his scores in Betweeness Centrality, 14,784. Along the multiple paths to get from person to person across the network, Socrates lies right in the pathway to a very large extent, providing a short-cut through the network.[7] But as we can see in Figure 10, where the top twenty high scorers are selected, many people play this role of bridge or broker to connect people all around the network. Using SNA mainly involves searching for patterns. In Figure 10 there is more red at the top of the network than we saw in Degree Centrality (Fig. 9) which was mainly in the lower part.
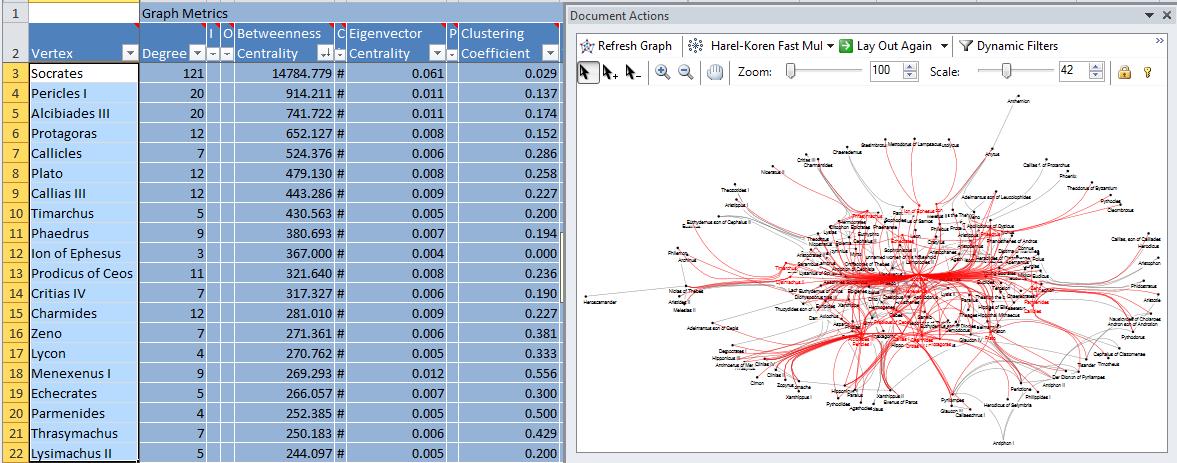
We next look at the Eigenvector Centrality column. This score doesn’t just count the number of ties, but weights them, so that we understand the extent to which any actor is tied to other people with high scores. It is often used as a rough way of judging someone’s prestige, or proximity to power. Socrates scores the highest of anyone, 0.061. In Figure 11 we see the top ten scorers in Eigenvector centrality, and we notice how closely they are hugging Socrates (his node was not selected so that we could see the others more clearly). They are mostly in the lower part towards the left in the sociogram. Comparing the three charts in Figures 9, 10, and 11 we see different people playing different roles inside the network, each of whom could be further studied. The statistics can lead to avenues for discovery.
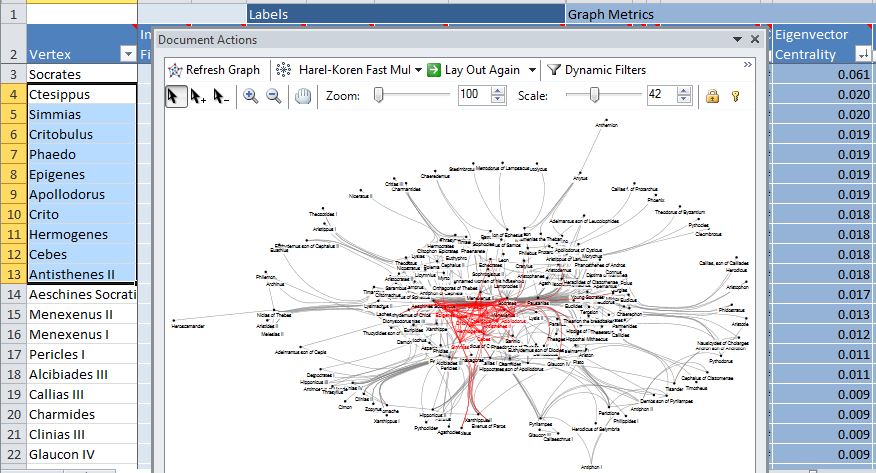
Now of all these measures, it seemed to me that if we could find the people who scored the highest in the Eigenvector centrality out of all 186 vertices with first degree ties, those would be the most likely to be Socrates’s most intimate associates. Before we find out the results from NodeXL, let’s look at some textual evidence.
This first passage that names the closest associates of Socrates is from Xenophon’s Memorabilia, 1.2.48:[8]
But Criton was a true associate of Socrates, as were Chaerophon, Chaerecrates, Hermogenes, Simmias, Cebes, Phaedondas, and others who consorted with him not that they might shine in the courts or the assembly, but that they might become gentlemen, and be able to do their duty by house and household, and relatives and friends, and city and citizens. Of these not one, in his youth or old age, did evil or incurred censure.
Most frustrating is the phrase “and others” which leaves the list incomplete.
A second quote comes from Plato’s Apology 33b-34a, put into Socrates’s mouth while on trial for his life.
[33b] But why then do some people love to spend much of their time with me? You have heard the reason, men of Athens; for I told you the whole truth; it is because they like to listen when those are examined who think they are wise and are not so; for it is amusing….[33d] And there are many of them present, whom I see; first Crito here, who is of my own age and my own deme and father of Critobulus, who is also present; then there is Lysanias the Sphettian, father of Aeschines, who is here; and also Antiphon of Cephisus, father of Epigenes. Then there are others whose brothers joined in my conversations, Nicostratus, son of Theozotides and brother of Theodotus (now Theodotus is dead, so he could not stop him by entreaties), and Paralus, son of Demodocus; Theages was his brother; and [34a] Adeimantus, son of Aristo, whose brother is Plato here; and Aeantodorus, whose brother Apollodorus is present. And I can mention to you many others…[9]
The third relevant passage comes from Diogenes Laertius’s Lives of the Eminent Philosophers 2.5.47.
Of those who succeeded him and were called Socratics, the chief were Plato, Xenophon, Antisthenes, and of ten names on the traditional list the most distinguished are Aeschines, Phaedo, Euclides, Aristippus. I must first speak of Xenophon; Antisthenes will come afterwards among the Cynics; after Xenophon I shall take the Socratics proper, and so pass on to Plato. With Plato the ten schools begin: he was himself the founder of the First Academy.
We wish we knew the “ten on the traditional list” but those are lost. This passage focuses on the philosophers who were closest to Socrates and carried on his work, not necessarily the most intimate with him socially during his life.
We can compare these lists of names side by side in a spreadsheet (Figure 12), and then add a column with the list of people with the highest Eigenvector scores as generated by the social network analysis. Of the top thirteen with the highest Eigenvector Centrality scores, all but three names also appear in one of the other lists. The three that are unique to the Eigenvector list are each very interesting cases. Ctesippus, for example, is present in three of Plato’s dialogues, and Debra Nails s.v. Ctesippus notes that it is likely he is someone Plato knew and was in Socrates’s inner circle.[10] Menexenus is the interlocutor in the dialogue of the same name. And Alcibiades (as well as Critobulus) were known to be enamored with or intimate with Socrates.[11]
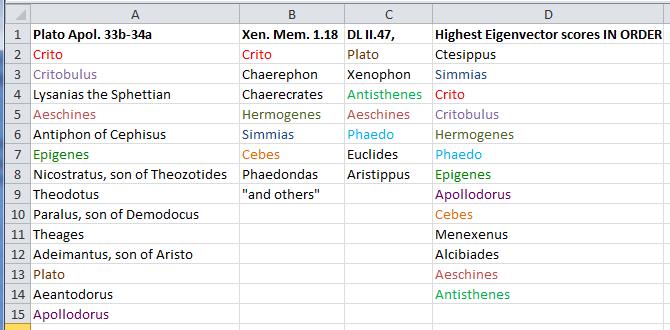
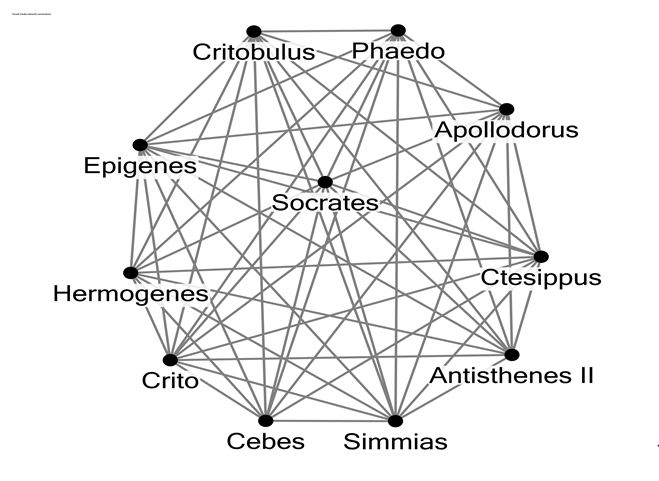
In Figure 13 we have the people with the highest Eigenvector scores, and it turns out they also know each other, forming a very large clique. They are the core of the group called the Socratics, consisting of current and former students and close friends. Many of them appear in Plato’s dialogues, and were present by Socrates’s bedside at his death. Ctesippus is the anomaly here, scoring the highest of all of them (Figure 11); Nails noted that we know little about him, not even his patronymic. She also observed that he connects two groups of people in two dialogues, Lysis and Euthydemus. In SNA terms, this makes him a bridge inside the network connecting two clusters, and elevates his eigenvector score because of knowing more people with high scores.
Socrates’s Other Relationships
We have identified those whose scores suggest they are the most intimate with Socrates, but perhaps it is more important to see how he mingled and crossed over into all sorts of communities. In public, Socrates was famous for going to the center of the city, the Agora, and talking to people, with a following of young people observing, listening and sometimes laughing. This is one of the reasons that he was charged with corrupting the youth and tried in court in 399 BC. Take this example from a passage in Xenophon’s Memorabilia:[12]
Moreover, Socrates lived ever in the open; for early in the morning he went to the public promenades and training-grounds; in the forenoon he was seen in the market; and the rest of the day he passed just where most people were to be met: he was generally talking, and anyone might listen. Xen. Mem. 1.10
The settings just described are places where Socrates had a primary interlocutor, a shoe-maker like Simon[13], say, but where others could observe and listen and learn indirectly. Figure 14 shows just how many people Socrates associated with who were not intellectuals or philosophers, and the large circle on the left are people who fell into that category.
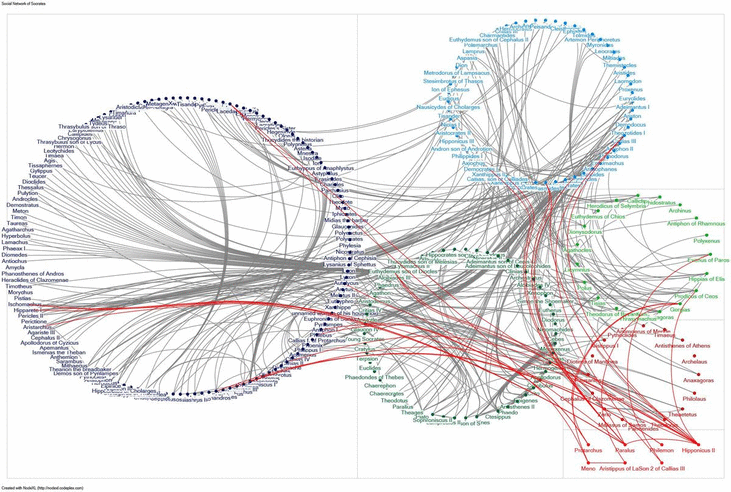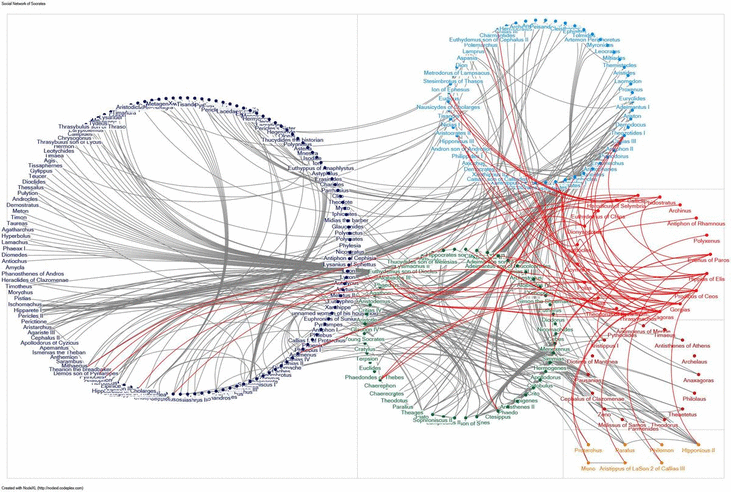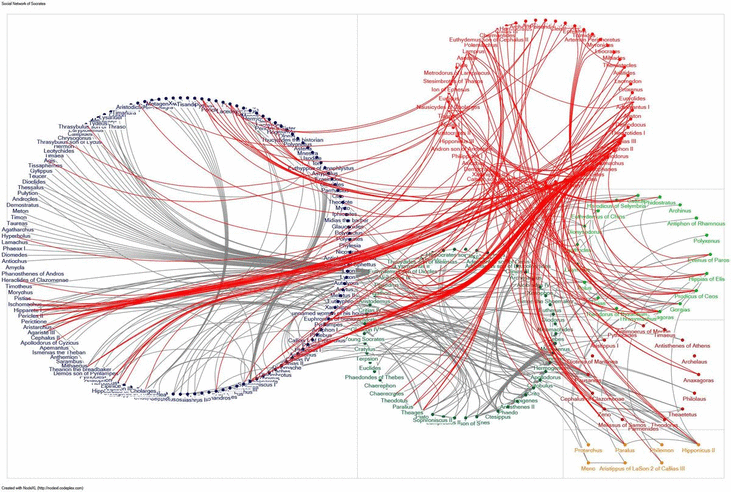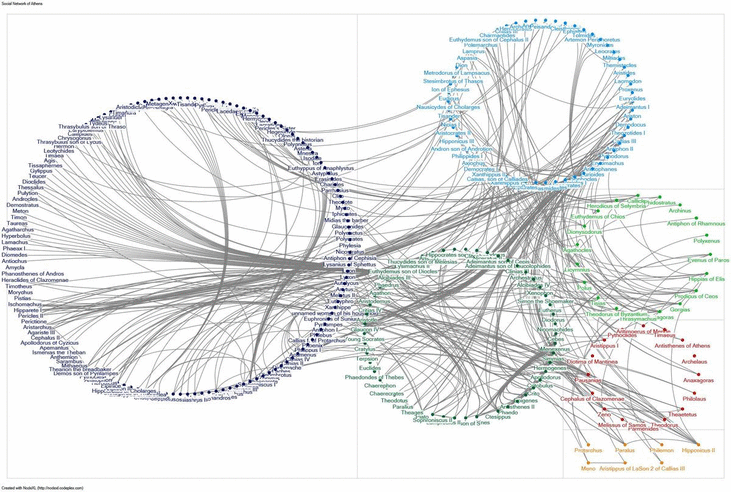
In the chart in Figure 14 we see Socrates in the lower center in a circle among his friends and followers, the Socratics. Each circle here is based not on a cluster analysis but on my attribute list, representing different roles. Figure 15 excludes Socrates’s ties, as he crowds out the ability to study everyone else’s relationships. From the lowest right corner in yellow are some students (of teachers other than Socrates), then a circle just above them on the far right in red consists of foreign philosophers, and above them in green are the sophists, and then the Socratics in dark green appear in the lower center. In the top right circle in light blue are intellectuals, people like Callias, the rich host of the symposium whom we met at the beginning, or people who were statesmen like Pericles or men who taught subjects other than oratory or philosophy. In the largest circle on the left in blue are the people with whom Socrates associated, who were not any of those things, and to our surprise it forms the largest component. One might say they are everyday people. When Socrates introduced people to each other, he was generating pathways for the flow of information. He served as the community’s connector, facilitator, and network weaver, or bridge and broker in Social Network Analysis terms, helping the network reach its maximally efficient state.
Conclusion
With Social Network Analysis, it is exciting to be able to visualize the invisible, to look beyond the familiar, and to ask questions that couldn’t be answered before. Social Network Analysis is a tool that is only recently making its way into the digital humanities, but it is already clear that it offers us opportunities to think differently about the ancient world.
Socrates introduced the elenchus, or the art of dialogue, to philosophy and education in the mid-fifth century BC. There is something about dialogue that is very social, that requires two interlocutors who create a link by engaging in this special kind of conversation. Plato in Meno 80d has Socrates say, “‘I want to examine and seek together with you what it might be.” Philosophical inquiry is an inherently social act for Socrates. He could not philosophize alone.
Bibliography
Borgatti, S. P., M.G. Everett, and J.C. Johnson. 2013. Analyzing Social Networks. London.
Cherven, K. 2013. Network Graph Analysis and Visualization with Gephi. Birmingham.
Cline, D. H. 2012. “Six Degrees of Alexander: Social Network Analysis as a Tool for Ancient History.” Ancient History Bulletin 26.1-2:59-70.
Dakyns, H. G. 1897. The Works of Xenophon. London.
Hamilton, E., and H. Cairns. 1961. The Collected Dialogues of Plato, Including the Letters. Princeton. Repr. 1980.
Hansen, D., B. Shneiderman, and M.A. Smith. 2011. Analyzing Social Media Networks with NodeXL: Insights from a Connected World. Burlington, MA.
Nails, D. 2002. The People of Plato: A Prosopography of Plato and Other Socratics. Indianapolis.
Nails, D. 2017. The People of Plato: A Prosopography of Plato and Other Socratic: Errata and Addenda, January 2017 downloaded from https://msu.edu/~nails/pop.pdf on December 28, 2018.
Newman, M. 2010. Networks: An Introduction. Oxford.
Wasserman, S., and K. Faust. 1994. Social Network Analysis: Methods and Applications. Cambridge.
Xenophon. 1923. Trans. E. C. Marchant. Cambridge, MA. 1923, downloaded from https://ebooks.adelaide.edu.au/x/xenophon/x5sy/chapter1 on December 15, 2018.
[1] I wish to thank the Center for Hellenic Studies, and G. Nagy and L. Muellner in particular, for their encouragement and their support of this project. Lia Hanhardt, the Editorial Assistant at CHS, worked on the project. Student assistants helped to check the data were invaluable to me and included Peri Buch and Adam Schilt. I am also grateful for the Fellowship in Hellenic Studies for 2018-19. George Washington University’s University Facilitating Fund provided some financial support. I thank Marijana Ricl for reviewing a draft; all errors remain my own.
[2] For an introduction to Social Network Analysis using the three main software programs, see Hansen et al 2011 for NodeXL; Cherven 2013 for Gephi; and Borgatti et al 2013 for UCInet.
[3] Nails 2002.
[4] Hansen et al. 2011: 35, 55.
[5] Text derived from Dakyns 1897.
[6] For more examples, see Cline 2012.
[7] Borgatti et al. 2013: 174; Newman 2010: 185, Wasserman and Faust 1994: 189.
[8] Xen. Sym. http://www.perseus.tufts.edu/hopper/text?doc=Xen.+Mem.+1.1.1&redirect=true
[9] Translated by Hamilton and Cairns 1961.
[10] Nails 2002: 119-120.
[11] Nails 2002: 20-22.
[12] Xenophon. Translated by E. C. Marchant.
[13] D. L. 2.122-3 discussed by Nails 2002: 261-262.